
Solve this, and you have the x -coordinate of the intersection.
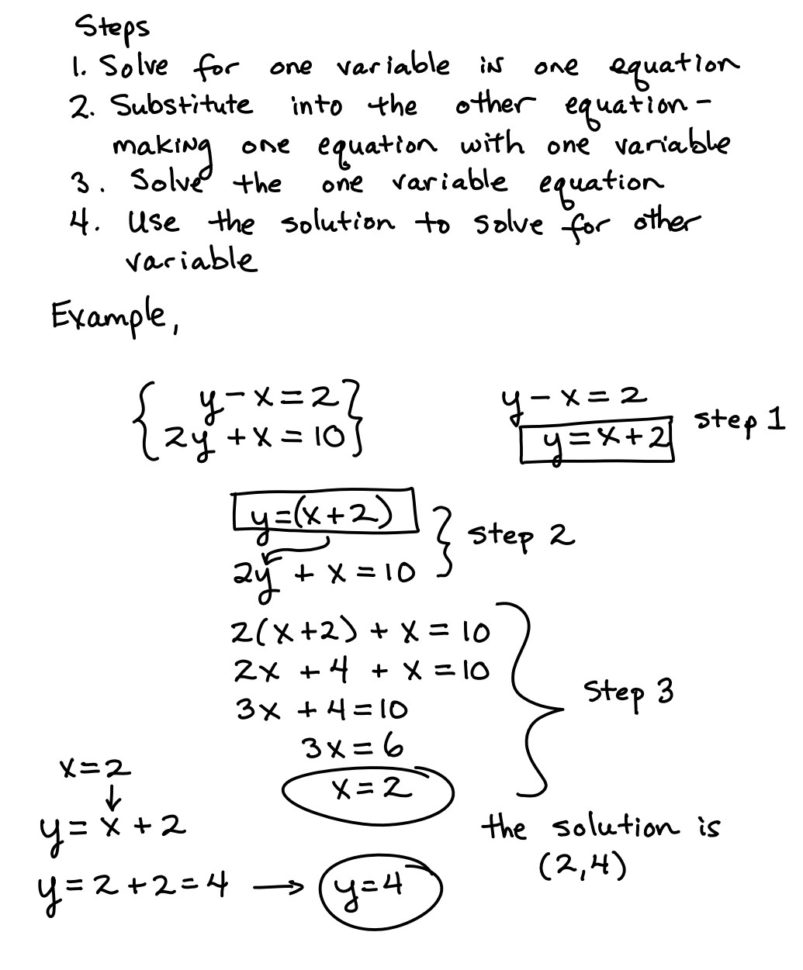
Step 2 : Then substitute that expression for y in the other linear equation. Steps for Using the Substitution Method in order to Solve Systems of Equations, Solve 1 equation for 1 variable. Step 1 : First, solve one linear equation for y in terms of x. Once an expression for the variable is found, substitute or plug in the expression into the other equation where the original variable was to solve for the. A system of two equations in x and y can be solved by rearranging one equation to represent x in terms of y, and then substituting this expression for x into. (The two equations represent the same line.) The lines intersect at infinitely many points. Steps for Using the Substitution Method in order to Solve Systems of Equations Solve 1 equation for 1 variable. The lines intersect at exactly one point. Systems of Equations: Substitution, Simultaneous, Cramers Rule: Algebra Practice Workbook with Answers (Improve Your Math Fluency) solving two equations with. How do you solve an equation with two substitutions?Ī system of linear equations is just a set of two or more linear equations.Īnd y ), the graph of a system of two equations is a pair of lines in the plane. Linear Systems with Infinitely Many Solutions. substitute) that variable in the other equation(s). Solving a Linear System using the Substitution Method Solving By Substitution Write one of the equations so it is in the style variable. 
Substitute the relation into the other equation: ( 3 + y ) + y = 5 ⇒ 3 + 2 y = 5 (3 + y ) + y = 5 \Rightarrow 3 + 2y = 5 ( 3 + y ) + y = 5 ⇒ 3 + 2 y = 5. Step 1: Isolate variable x x x using the second equation: x = 3 + y x = 3 + y x = 3 + y. If the equations are given in standard form, we’ll need to start by solving for one of the variables. Copy of Systems of equations (substitution method) 1) Solve one equation for one variable 2) Substitute the expression for the variable in other equation and.

Let's follow the above steps to solve the following system of equations: substitute the solved value back into the relations.repeat until we are left with a single variable, and solve for it.substitute the relation into the other equation(s) to reduce the number of variables by 1.
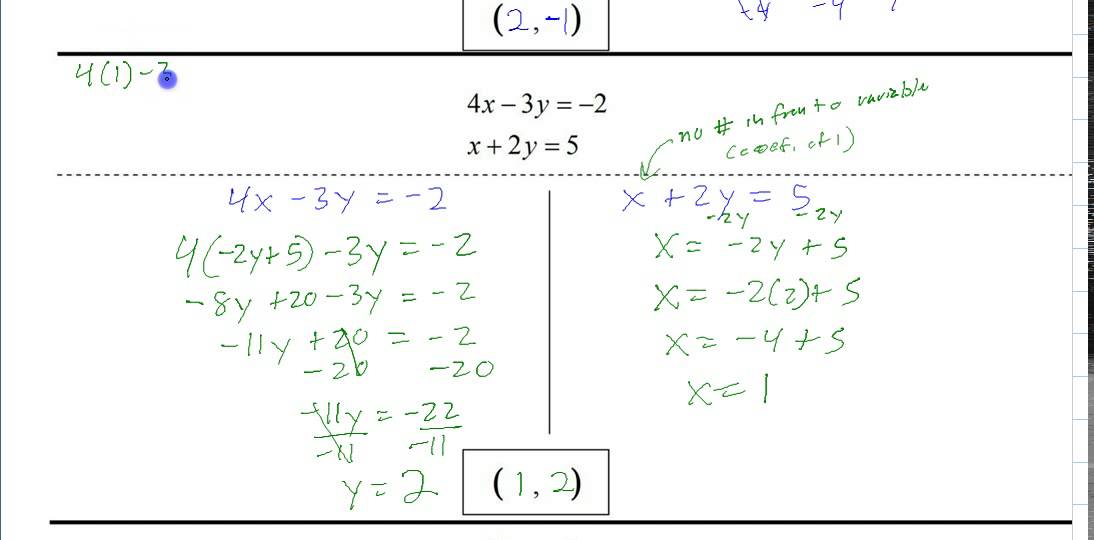
Then we substitute that value in the second equation. In this lesson, we are going to talk you through solving a system of 2 linear equations in 2 variables. The method can also be used to find the solution of a system of three or more equations in three or more variables, but it takes longer. In this method, we find the value of any one of the variables by isolating it on one side and taking every other term on the other side of the equation. Substitution is the quickest method of solving a system of two equations in two variables.
find a relation that isolates one of the variables by changing the subject One of the methods to solve a system of linear equations in two variables algebraically is the substitution method.








 0 kommentar(er)
0 kommentar(er)
